It often happens that two or more waves pass simultaneously through the same region. When we listen to a concert, for example, sound waves from many instruments fall simultaneously on our eardrums. The electrons in the antennas of our radio and television receivers are set in motion by the net effect of many electromagnetic waves from many different broadcasting centers. The water of a lake or harbor may be churned up by waves in the wakes of many boats.
Suppose that two waves travel simultaneously along the same stretched string. Let y1(x, t) and y2(x, t) be the displacements that the string would experience if each wave traveled alone. The displacement of the string when the waves overlap is then the algebraic sum
This summation of displacements along the string means that
Overlapping waves algebraically add to produce a resultant wave (or net wave).
This is another example of the principle of superposition, which says that when several effects occur simultaneously, their net effect is the sum of the individual effects.
Figure 16-14 shows a sequence of snapshots of two pulses traveling in opposite directions on the same stretched string. When the pulses overlap, the resultant pulse is their sum. Moreover, each pulse moves through the other as if the other were not present:
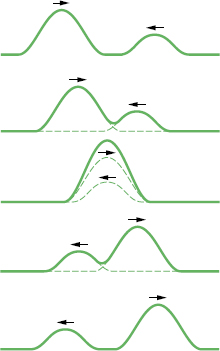
Fig. 16-14 A series of snapshots that show two pulses traveling in opposite directions along a stretched string. The superposition principle applies as the pulses move through each other.
Overlapping waves do not in any way alter the travel of each other.
Leave a Reply